Embibe Experts Solutions for Chapter: Simple Harmonic Motion, Exercise 1: Exercise 1

A block of mass $\text{'}m\text{'}$ is suspended from a spring and executes vertical $\mathrm{SHM}$ of time period $T$ as shown in figure. The amplitude of the $\mathrm{SHM}$ is $A$ and spring is never in compressed state during the oscillation. The magnitude of the minimum force exerted by spring on the block is

A horizontal rod of mass $m$ and length $L$ is pivoted smoothly at one end. The rod's other end is supported by a spring of force constant $k.$ The rod is rotated (in vertical plane) by a small angle $\theta$ from its horizontal equilibrium position and released.

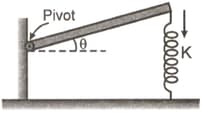
The angular frequency of the subsequent simple harmonic motion is:

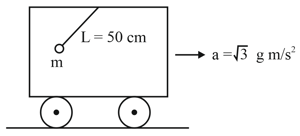
A simple pendulum $50 \mathrm{cm}$ long is suspended from the roof of a cart accelerating in the horizontal direction with constant acceleration $\sqrt{3} g \mathrm{m} {\mathrm{s}}^{-2}.$ The period of small oscillations of the pendulum about its equilibrium position is $\left(g={\mathrm{\pi }}^2 \mathrm{m} {\mathrm{s}}^{-2}\right)$:

A metre stick swinging in a vertical plane about a fixed horizontal axis passing through its one end undergoes a small oscillation of frequency $f_0.$ If the bottom half of the stick is cut off, then its new frequency of small oscillation would become:

Two particle of mass $m$ each are fixed to a massless rod of length $2\ell .$ The rod is smoothly hinged at one end to a ceiling. It performs oscillation of small-angle in vertical plane. The length of the equivalent simple pendulum is

A uniform disc of mass $m$ and radius $R$ is pivoted at its centre $O$ with its plane vertical as shown in the figure. A circular portion of the disc of radius $\frac{R}{2}$ is removed from it. The time period of small oscillations of remaining portion about $O$ is-

A disc is hinged such that it can freely rotate in a vertical plane about a point on its radius. If radius of disc is $R,$ then what will be minimum time period of its simple harmonic motion?

$x=x_1+x_2$ (where $x_1=4\cos \omega t$ and $\left.x_2=3\sin \omega t\right)$ is the equation of motion of a particle along $x$-axis. The phase difference between $x_1$ and $x$ is,